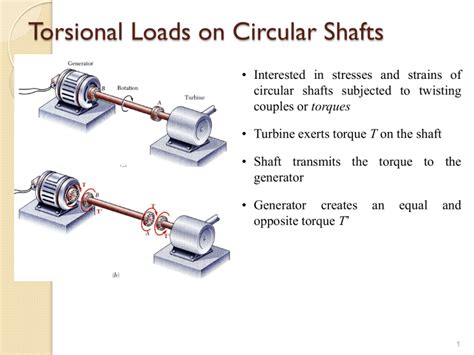
torsion test for circular cross section bars|shaft torsion diagram

20241127 | By thickness measuring shaft , DOD News

torsion test for circular cross section bars|shaft torsion diagram : discounter Upon completion of this chapter, you will be able to calculate the torque, or twisting moment, on a circular section of a shaft and to calculate the torsional shearing stresses . Loto - Quinielas en vivo,quinielas de hoy,resultados en direct.

{plog:ftitle_list}

Resultado da 8 de out. de 2009 · REMASTERED IN HD!Official Music Video for Chiquitita performed by ABBA.Listen to the new album: https://abba.lnk.to/VoyageAlbumListen .

I.1 To become familiar with torsion tests of rods with solid circular cross sections. I.2 To observe the relation between shear stress (τ) and shear strain (γ). I.3 To experimentally .Torsion of Circular Bar • From consideration of symmetry: – Cross sections of the circular bar rotates as rigid bodies about the longitudinal axis – Cross sections remain straight and circularConcept Question 6.3.1. Torsion of an elliptical bar Consider a bar with elliptical cross section as shown in the gure subject to a torque T at its ends. The boundary is described by the implicit .Torsion test is a method used to determine a material’s torsional stiffness and shear modulus for bars with circular cross sections. When a torsional force is applied to a bar the torque can be calculated and angle of twist is monitored, .

consider a bar or shaft of circular cross section twisted by a couple T, assume the left-hand end is fixed and the right-hand end will rotate a small angle. , called angle of twist. if every cross .

Upon completion of this chapter, you will be able to calculate the torque, or twisting moment, on a circular section of a shaft and to calculate the torsional shearing stresses .Torsion Test on Mild Steel. In torsion testing the circular bar is placed in the machine such a way that its longitudinal axis coincides with the axis of the grips and so that it remains straight during the test.

Unlike axial loads which produce a uniform, or average, stress over the cross section of the object, a torque creates a distribution of stress over the cross section. To keep things .(1) This is an equation that shows the ability of a circular cross-section beam or specimen to resist torsion (twisting). A higher polar moment of inertia shows that the beam or specimen can resist .

torsional testing of shafts

Circular beams are further divided into those with uniform cross sections (Section 1.5.1.1) and those with nonuniform cross sections (Section 1.5.1.2). Noncircular beams are divided into open noncircular beams (Section 1.5.2.1) .1. bar have circular cross section (either solid or hollow) 2. material is linear elastic note that the above equations cannot be used for bars of noncircular shapes, because their cross sections do not remain plane and their maximum stresses are not located at the farthest distances from the midpoint Example 3-1 The test specimens were 6061-T6 and 5050-H32 alloys with solid circular and solid rectangular cross-sections. The test plan included torsion tests until rupture, for 5 sets of 6061-T6 specimens with circular and rectangular cross-sections, and 4 sets of 5050-H32 specimens with rectangular cross-sections.

For example torsion of a bar with cross-section that can be split into various rectangles. Analysis of shear stress of the particular rectangles . non-circular bar, the real cross-sections are deﬂected from the planar shape. The equation for a non-circular bar is derived correctly in [7], but no solutions for particular proﬁles are

shear stress on any cross section. Failure might occur due to shear alone or because the shear is accompanied by stretching or bending. Objective: To determine Shear Modulus of Elasticity (G) of some Steel, Aluminum and Brass circular shafts and develop a relationship among the Torque (T0 and Clamping length (L) and the angle of twist (θ )

torsional testing of shafts

A narrow cross-sectional beam is a rectangular beam that has a width that is much greater then its thickness. Since the cross-section is not circular the stress will vary on the outside. Which can be seen in the stress profile below. Notice also that the higher stress concentration is located at the end in the center where there would normally . In the simplest case, the torsion bar can be defined as a prismatic body with constant circular cross-section (outside radius \(R\)) and constant shear modulus \(G\), which is loaded with a torsional moment \(M\) in the direction of the body axis. Figure 4.1a illustrates the torsion bar with applied load and Fig. 4.1b shows the free body diagram.Torsion equation or torsion constant is defined as the geometrical property of a bar’s cross-section that is involved in the axis of the bar that has a relationship between the angle of twist . The circular section should be circular. The stress of the material should not exceed the elastic limit. Test your knowledge on Torsion equation . the bar or shaft is circular in cross-section and prismatic, which means it has the same cross-section shape throughout its length. . Fig 7. Cross-section of a bar in pure torsion showing an element of area d A \mathrm{d} . Now we can test what the maximum torque would be if the angle of twist were the limiting criteria using, T = .

Download Citation | Torsion in Circular Sections | When a circular bar is subjected to a twisting action it tends to twist; the stresses developed in the bar are called torsional stresses. In .Lab#2 Torsion experiment torsion test of metallic materials course section: mech date performed: 16th june 2021 group number: : gissel perez siddhant nayak . Torsion Test of Metallic Materials Course Section: MECH 237-Date Performed: 16th June 2021 . illustrate the bar once it's twisted. Then, we also need to take into consideration .Circular shaft experiencing an axial torque. A solid, circular cross-sectioned shaft experiences an axial torque T, as shown above.. Angle of twist The hypothesis used in developing the stress and strain in the shaft is that all points on a cross-section of the shaft experience the same angle of .The objectives of this experiment are to perform a torsion test of a steel bar, examine the strain and stress distribution due to torsion in a bar with a circular cross-section, experimentally determine the shearing modulus of steel, and observe the failure mode of a ductile material in torsion. B. Theory. Equilibrium

I p - Polar moment of inertia of the bar cross-section. In the general case, the maximum shear stresses occur at the edge of the cross-section, except for the outer corners, where the shear stresses are equal to zero. A bar of non-circular cross-section undergoes warping where the points of its cross-section go out of the plane and move along .2.3 Uniform Torsion in a Circular Cross Section Let us consider a bar of constant circular cross section subjected to torsion as shown in Fig. 1. In this case, plane cross sections normal to the axis of the member remain plane after twisting, i.e. there is no warping. The torque is solely resisted by circumferentialFigure 9.7: Shear stresses in a bar with solid circular cross section subjected to end torsion. Then, the angle of twist per unit length, Ω is also obtained from as, (9.19) . Here we study the problem of end torsion of a bar whose cross section is narrow and has small curvature such as those shown in figure 9.20. Such sections are usually .Formulas for bars of non - circular section. Bars of non -circular section tend to behave non-symmetrically when under torque and plane sections to not remain plane. Also the distribution of stress in a section is not necessarily linear. The general formula of torsional stiffness of bars of non-circular section are as shown below the factor J .

Torsion test is a method used to determine a material’s torsional stiffness and shear modulus for bars with circular cross sections. When a torsional force is applied to a bar the torque can be calculated and angle of twist is monitored, and these values are plotted on a graph in order to determine the torsional stiffness and shear modulus by .

CHAPTER 5 TORSION OF NON-CIRCULAR AND THIN-WALLED SECTIONS Summary For torsion of rectangular sections the maximum shear stress tmax and angle of twist 0 are given by T tmax = ~ kldb2 e - T L k2db3G kl and k2 being two constants, their values depending on the ratio dlb and being given in Table 5.1. For narrow rectangular sections, kl = k2 = i.The prismatic bar is 800 mm in length and has a circular cross section with 100-mm diameter. In a test to determine its elastic properties, the bar is subjected to 4.8-MN axial loads. After the loads are applied, the bar’s length is 802.44 mm, and its diameter is 99.902 mm. . Except for our analysis of thin-walled cross sections in Sect. 4. .3) The cross-sectional planes of a specimen tested in torsion remain plane during testing of non-circular cross-sections. True/False. 4) From a torsion test, the slope of the initial straight part of the shear-stress-shear-strain diagram is . A. Young's Modulus. B. The shear modulus, E. C. The modulus of rigidty, G

The objectives of the torsion experiment include determination of shear modulus of elasticity “G” and shear proportional limit “τp” of the material. In the torsion test, a torque “T” is applied to one end of a circular cross-section metallic rod while the other end is held fixed in a stationary grip. For a circular cross-section, it’s typically τ = T / J, where τ is torsional stress, T is torsional moment, and J is the polar moment of inertia. How do you calculate torsion test? A torsion test involves applying torque to a specimen and measuring its deformation. The calculation of torsion test results depends on the specific test setup .

Torsion of Prismatic Bars of Circular Sections Required assumptions to determine the deformation of a shaft and internal shearing stresses. Cross sections of perpendicular to the longitudinal axis of the shaft are plane prior and after the .Concept Question 6.3.1. Torsion of an elliptical bar Consider a bar with elliptical cross section as shown in the gure subject to a torque T at its ends. The boundary is described by the implicit equation f(x 1;x 2) = x 2 1 a 2 + x 2 b 1 = 0 a b x 1 x 2 Figure 6.4: Elliptical cross-section. 1.Propose a functional form for the stress function .The Torsion constant is considered as a geometrical property of the cross-section of a bar that exists in the relationship between the applied torque along with the axis’s bar and the angle of twist. All this theory is applied to a homogeneous plastic bar. . This process is also termed as the Derivation of the Torsion Equation for a .

Bauer Fiber Classifier Φ100 convenience store

torsional testing of circular shaft